Welcome to our simple guide to converting RPM to Rad/s. Whether you’re an engineer, physicist, or just someone curious about the topic, this guide is designed to help you easily understand how to convert RPM to Rad/s. As a Canadian, it’s important to know this conversion as it is used in various industries such as manufacturing, automotive, and aviation.
First, let’s briefly discuss what RPM and Rad/s are and their relationship to each other. Then, we’ll provide you with the conversion formula and tips for achieving accuracy when converting RPM to Rad/s. We’ll also show you some examples of how to convert RPM to Rad/s with step-by-step calculations. Finally, we’ll discuss some common applications of this conversion and the benefits of using a conversion calculator.
By the end of this guide, you’ll have a clear understanding of how to easily convert RPM to Rad/s and why it’s important to do so accurately. Let’s get started!
Understanding RPM and Rad/s
Before we dive into the conversion process, it’s important to understand what RPM and Rad/s actually are. RPM, or Revolutions Per Minute, is a unit of measurement used to describe rotational speed. It represents the number of complete rotations that a rotating object makes in one minute.
On the other hand, Rad/s, or Radians Per Second, is a unit of angular velocity. A radian is a unit of angle measurement, defined as the angle subtended at the center of a circle by an arc of its circumference equal in length to the radius of the circle. So, Rad/s represents the angular speed of an object in radians per second.
The key difference between RPM and Rad/s is that RPM is a measure of linear velocity, while Rad/s is a measure of angular velocity. Therefore, converting between the two requires a specific formula to account for this difference.
Relationship between RPM and Rad/s
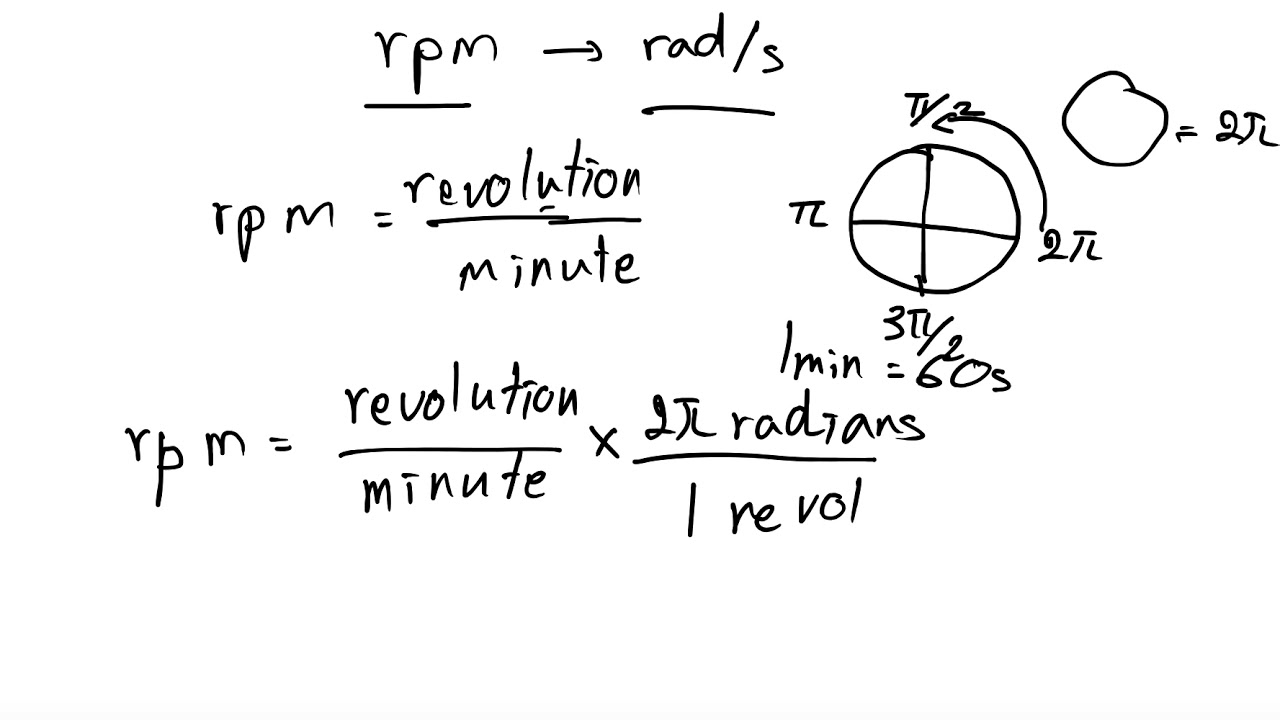
To better understand the relationship between these two units, consider the following: one revolution around a circle is equal to 2π radians. Therefore, to convert RPM to Rad/s, we need to multiply the RPM value by 2π/60.
| RPM | Rad/s |
|---|---|
| 1 | 0.10472 |
| 10 | 1.0472 |
| 100 | 10.472 |
For example, if a motor is running at 1000 RPM, the angular velocity can be calculated as follows:
Rad/s = RPM x 2π/60 = 1000 x 2π/60 = 104.72 Rad/s
It’s important to note that the direction of rotation doesn’t affect the conversion factor, as long as the same direction is used consistently throughout the calculation.
Now that we have a better understanding of RPM and Rad/s, let’s move on to the conversion process and how to use the formula.
Conversion Formula and Calculation
Converting RPM to Rad/s may seem daunting at first, but it’s actually quite simple. The conversion formula is:
Rad/s = RPM x (π/30)
Let’s break this down into steps:
- Write down the value of RPM you want to convert.
- Multiply the value by π (pi).
- Divide the result by 30.
Here’s an example:
Example: Convert 1200 RPM to Rad/s.
Solution:
| RPM | (π/30) | Rad/s | ||
|---|---|---|---|---|
| 1200 | x | 0.104719755 | = | 125.6637061 |
Therefore, 1200 RPM is equivalent to 125.66 Rad/s (rounded to two decimal places).
It’s important to note that this formula assumes that the rotation axis is constant and that the conversion is instantaneous. However, in reality, the rotation axis may change or the conversion may take time. In such cases, it’s best to consult a qualified engineer or use specialized equipment to measure the angular velocity precisely.
If you prefer a quicker method, you can use a conversion calculator. Many online calculators are available for free, and some even feature additional functions like unit conversions and error analysis. Enter the RPM value, and the calculator will automatically convert it to Rad/s for you.
Tips for Converting RPM to Rad/s
Converting RPM to Rad/s is a crucial task that requires precision and accuracy to ensure accurate results. Here are some practical tips to keep in mind when performing this conversion:
- Remember the formula: The formula for converting RPM to Rad/s is simple: Rad/s = RPM x (2π/60). It’s essential to keep this formula in mind when performing the conversion.
- Convert units: Ensure that the units are consistent throughout the calculation. For instance, if the given RPM is in revolutions per minute, convert it to radians per second before plugging it into the formula.
- Round off: Decide the number of significant figures you require for your final answer and round it off accordingly. However, avoid rounding off prematurely before completing the entire calculation.
- Check your answer: Always double check your answer to ensure accuracy. If possible, compare it with an online RPM to Rad/s converter or calculator.
By keeping these tips in mind, you can ensure that your conversions are accurate and precise, resulting in reliable calculations.
Conversion Examples
Here are some conversion examples to clarify the process of converting RPM to Rad/s:
| RPM | Rad/s |
|---|---|
| 100 | 10.47 |
| 500 | 52.36 |
| 1000 | 104.72 |
Let’s take the first example of converting 100 RPM to Rad/s. To do this, we use the conversion formula:
Rad/s = RPM x π/30
Substituting 100 for RPM, we get:
Rad/s = 100 x π/30
Simplifying the equation gives us:
Rad/s = 10.47
Therefore, 100 RPM is equal to approximately 10.47 Rad/s.
This same process can be applied to convert any RPM value to Rad/s. Just remember to use the conversion formula and simplify the equation. Additionally, be mindful of rounding and significant figures when reporting your results.
For quick and accurate conversions, we recommend using a conversion calculator or smartphone app.
Common Applications of RPM to Rad/s Conversion
Converting RPM to Rad/s plays a vital role in various fields, including engineering, physics, and mechanics. Let’s explore some common applications:
- In engineering, converting RPM to Rad/s is necessary when designing and analyzing machines such as engines, turbines, and pumps. It helps engineers to precisely calculate rotational speed and torque, enabling them to optimize the efficiency and safety of these machines.
- In physics, the conversion is commonly used to measure and analyze rotational motion, including the motion of planets, galaxies, and atoms. It helps physicists to accurately determine angular velocity, angular acceleration, and other essential parameters in their experiments.
- In mechanics, the conversion is crucial for understanding and predicting the behavior of moving objects. It allows us to convert linear speed to angular speed, which is often required in the analysis of vehicles, aircraft, and other moving systems.
Overall, converting RPM to Rad/s has a wide range of practical applications in many fields, making it a valuable skill for Canadians pursuing careers in these areas.
Importance of Using a Conversion Calculator
When converting RPM to Rad/s, it’s essential to achieve accuracy and precision in your calculations. This is where conversion calculators come in handy.
Online tools or smartphone apps can perform instant conversions, saving time and reducing the likelihood of making errors. Using a calculator also allows you to focus on the more complex aspects of your work, ensuring you can complete it more efficiently.
Several calculators are available online that are reliable and user-friendly. They are equipped with advanced features such as the ability to convert multiple units simultaneously.
Ensure that you are using reputable and trustworthy calculators from reputable sources. Always verify the accuracy of the calculator by performing a few test calculations.
Overall, using a conversion calculator can help you complete your work more efficiently and accurately. It makes the process of converting RPM to Rad/s much simpler, allowing you to focus on other aspects of your project.
Additional Tips for Precision and Accuracy
When converting RPM to Rad/s, it’s important to strive for precision and accuracy to ensure calculations are correct. Here are some additional tips to consider:
- Pay attention to rounding rules: When rounding numbers, consider the number of significant figures and the rounding rules for the final result. Incorrect rounding can lead to errors in the final answer.
- Use the correct number of decimal places: Make sure to use the correct number of decimal places for the final answer, depending on the required precision. Using too many or too few decimal places can also lead to errors.
- Consider error margins: Keep in mind that all measurements have a degree of uncertainty or error. It’s important to consider this when performing calculations and be aware of the potential impact on the final result.
- Avoid unit conversions: When converting from RPM to Rad/s, it’s important to ensure that the units are consistent throughout the calculation. Avoid unnecessary conversions between units to minimize the risk of errors.
By following these tips, you can achieve accurate and precise conversions from RPM to Rad/s.
Conclusion
Converting RPM to Rad/s is an essential calculation used in various fields like engineering, physics, and mechanics. As we have seen, the process is straightforward, and with the right formula and calculator, it can be accomplished efficiently and accurately.
Remember to keep in mind the tips we shared for achieving precision and accuracy, such as rounding, significant figures, and error margins. It’s also crucial to understand the relationship between RPM and Rad/s and why we convert between the two.
Practice Makes Perfect
To ensure mastery of converting RPM to Rad/s, we encourage you to practice with the examples we provided and explore additional resources. The more familiar you become with the process, the more comfortable you will be applying it in real-life scenarios.
Finally, we recommend using online calculators and smartphone apps for quick and accurate conversions. This will save time and effort, allowing you to focus on other critical tasks. Remember to choose a reliable conversion calculator that fits your needs.
Thank you for reading our guide on converting RPM to Rad/s. We hope you found it helpful and informative. Happy converting!